de Broglie Wavelength

The energy of the electron, in the hydrogen atom, is known to be expressible in the form

$E_n=\frac{-13.6\text{ eV}}{n^2}\left(n=1,2,3,\mathrm{.....}\right).$

sing this expression, which of the following statement is/are true?

(i) Electron in the hydrogen atom cannot have energy of $-6.8\text{ eV}$ .

(ii) Spacing between the lines (consecutive energy levels) within the given set of the observed hydrogen spectrum decreases as n increases.

For what kinetic energy of a proton, will the associated de Broglie wavelength be 16.5 nm?

For what kinetic energy of a neutron the associated de Broglie wavelength be $1.32\times {10}^{-10} \mathrm{m}$?

An $\alpha -$ particle and a proton are accelerated from rest by the same potential. The ratio of their de Broglie wavelengths would be:

An $\alpha -$particle and a proton are accelerated from rest by the same potential. The ratio of their de Broglie wavelengths would be,

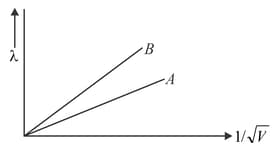
Two lines, A and B, in the plot given below show the variation of de Brogile wavelength,  $\lambda$  versus $1/\sqrt{V}$ , where V is the accelerating potential difference, for two particles carrying the same charge. Which one of two represents a particle of smaller mass?

The Davisson-Germer experiment demonstrated

de Broglie wavelength of an electron is $11\mathrm{nm}$. The energy of the electron is

Planks constant =$6.626\times {10}^{-34} \mathrm{J} \mathrm{s}$

Charge of electron=$1.6\times {10}^{-19} \mathrm{C}$

A neutron has a kinetic energy of $40\mathrm{meV}$$.$ Its de-Broglie wavelength is approximately

de Broglie wavelength of an electron having a kinetic energy of $13.6 \mathrm{eV}$ is $0.33 \mathrm{nm}.$ What would be the de Broglie wavelength if it had a kinetic energy of $3.4 \mathrm{eV}?$

Calculate the percentage change in the de-Broglie wavelength of the particle, if the kinetic energy of the particle is increased to $16$ times its previous value.

Find the ratio of kinetic energy of the electron to that of the energy of photon, if de Broglie wavelength of an electron moving with velocity $1.5\times {10}^8 \mathrm{m} {\mathrm{s}}^{-1}$, is equal to that of a photon.

A constant electric field $E$ accelerates a charged particle of mass $m$ and charge $e$, which was initially at rest. The rate of change of de-Broglie wavelength of this electron at time $t$ ignoring relativistic effects is:

From the following, moving with the same velocity, the one which has the largest wavelength:

The relativistic expression for the wavelength of electrons moving with very high speed is

A particle of charge, $q$ and mass, $m$ enters a region of a transverse electric field of, $E_0 \hat{\mathrm{j}}$ with an initial velocity, $v_0\hat{\mathrm{i}}$. The time taken for the change in the de-Broglie wavelength of the charge from the initial value of ${\lambda }_0$ to $\frac{{\lambda }_0}{3}$ is proportional to,

Davisson and Germer experiment was the experimental discovery of :